Dimensions and Units
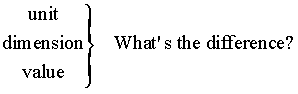
Many people aren’t sure of the difference. Let’s try and get a set of definitions we can use. Consider
- 110 mg of sodium
- 24 hands high
- 5 gal of gasoline
We’ll break them up this way:
| Value | Unit | Dimension |
| 110 | mg | mass |
| 24 | hand | length |
| 5 | gal | volume (length3) |
A “dimension” can be measured or derived. The “fundamental dimensions” (length, time, mass, temperature, amount) are distinct and are sufficient to define all the others. We also use many derived dimensions (velocity, volume, density, etc.) for convenience.
“Units” can be counted or measured. Measured units are specific values of dimensions defined by law or custom. Many different units can be used for a single dimension, as inches, miles, centimeters, furlongs, and versts are all units used to measure the dimension length.
Units and Calculations
It is always good practice to attach units to all numbers in an engineering calculation. Doing so
- attaches physical meaning to the numbers used,
- gives clues to methods for how the problem should be solved, and
- reduces the possibility of accidentally inverting part of the calculation.
Addition and Subtraction
- Values MAY be added if UNITS are the same.
- Values CANNOT be added if DIMENSIONS are different.
EXAMPLES:
different dimensions: length, temperature — so cannot be added
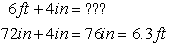same dimension: length, different units — can add
Multiplication and Division
Values may be combined; units combine in similar fashion.
EXAMPLES:
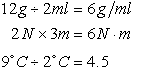
4.5 is a “dimensionless” quantity (in this case a pure number)
You cannot cancel or lump units unless they are identical.
Functions
Trigonometric functions can only have angular units (radians, degrees). All other functions and function arguments, including exponentiation, powers, etc., must be dimensionless.
EXAMPLES:
is OK; but 
is meaningless.
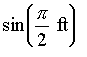is never defined.
Dimensional Homogeneity
Every valid equation must be “dimensionally homogeneous” (a.k.a. dimensionally consistent). All additive terms must have the same dimension.
Consider an equation from physics that describes the position of a moving object:
length [=] length + velocity*time + acceleration*time2
[=] length + (length/time)*time + (length/time2)*time2
[=] length + length + length
so the equation is dimensionally homogeneous. But just because an equation is homogeneous, doesn’t mean that it is valid!
- Not consistent –> Not Valid
- Consistent -\-> Valid
Dimensionless Quantities
When we say a quantity is dimensionless, we mean one of two things. First, it may just be a number like we get when counting.
EXAMPLES: Dimensionless Numbers
- Pi is a dimensionless number representing the ratio of the circumference of a circle to its diameter
- 2 prunes (counted)
Second — and of particular interest in engineering — are combinations of variables where all the dimension/units have “canceled out” so that the net term has no dimension.
These are often called “dimensionless groups” or “dimensionless numbers” and often have special names and meanings. Most of these have been found using techniques of “dimensional analysis” — a way of examining physical phenomena by looking at the dimensions that occur in the problem without considering any numbers.
Probably the most common dimensionless group used in chemical engineering is the “Reynolds Number”, given by
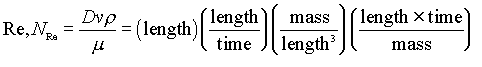
This describes the ratio of inertial forces to viscous forces (or convective momentum transport to molecular momentum transport) in a flowing fluid. It thus serves to indicate the degree of turbulence. Low Reynolds numbers mean the fluid flows in “lamina” (layers), while high values mean the flow has many turbulent eddies.
Units & Dimensions
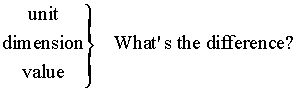
Many people aren’t sure of the difference. Let’s try and get a set of definitions we can use. Consider
- 110 mg of sodium
- 24 hands high
- 5 gal of gasoline
We’ll break them up this way:
| Value | Unit | Dimension |
| 110 | mg | mass |
| 24 | hand | length |
| 5 | gal | volume (length3) |
A “dimension” can be measured or derived. The “fundamental dimensions” (length, time, mass, temperature, amount) are distinct and are sufficient to define all the others. We also use many derived dimensions (velocity, volume, density, etc.) for convenience.
“Units” can be counted or measured. Measured units are specific values of dimensions defined by law or custom. Many different units can be used for a single dimension, as inches, miles, centimeters, furlongs, and versts are all units used to measure the dimension length.
Units and Calculations
It is always good practice to attach units to all numbers in an engineering calculation. Doing so
- attaches physical meaning to the numbers used,
- gives clues to methods for how the problem should be solved, and
- reduces the possibility of accidentally inverting part of the calculation.
Addition and Subtraction
- Values MAY be added if UNITS are the same.
- Values CANNOT be added if DIMENSIONS are different.
EXAMPLES:
different dimensions: length, temperature — so cannot be added
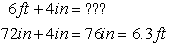same dimension: length, different units — can add
Multiplication and Division
Values may be combined; units combine in similar fashion.
EXAMPLES:
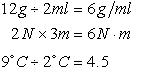
4.5 is a “dimensionless” quantity (in this case a pure number)
You cannot cancel or lump units unless they are identical.
Functions
Trigonometric functions can only have angular units (radians, degrees). All other functions and function arguments, including exponentiation, powers, etc., must be dimensionless.
EXAMPLES:
is OK; but 
is meaningless.
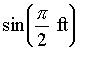is never defined.
Dimensional Homogeneity
Every valid equation must be “dimensionally homogeneous” (a.k.a. dimensionally consistent). All additive terms must have the same dimension.
Consider an equation from physics that describes the position of a moving object:
length [=] length + velocity*time + acceleration*time2
[=] length + (length/time)*time + (length/time2)*time2
[=] length + length + length
so the equation is dimensionally homogeneous. But just because an equation is homogeneous, doesn’t mean that it is valid!
- Not consistent –> Not Valid
- Consistent -\-> Valid
Dimensionless Quantities
When we say a quantity is dimensionless, we mean one of two things. First, it may just be a number like we get when counting.
EXAMPLES: Dimensionless Numbers
- Pi is a dimensionless number representing the ratio of the circumference of a circle to its diameter
- 2 prunes (counted)
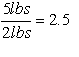
Second — and of particular interest in engineering — are combinations of variables where all the dimension/units have “canceled out” so that the net term has no dimension.
These are often called “dimensionless groups” or “dimensionless numbers” and often have special names and meanings. Most of these have been found using techniques of “dimensional analysis” — a way of examining physical phenomena by looking at the dimensions that occur in the problem without considering any numbers.
Probably the most common dimensionless group used in chemical engineering is the “Reynolds Number”, given by
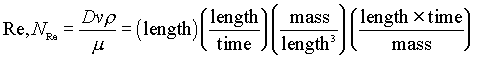
This describes the ratio of inertial forces to viscous forces (or convective momentum transport to molecular momentum transport) in a flowing fluid. It thus serves to indicate the degree of turbulence. Low Reynolds numbers mean the fluid flows in “lamina” (layers), while high values mean the flow has many turbulent eddies.