Centre of mass
Every body is a collection of large number of tiny particles. In translatory motion of a body, every particle experiences equal displacement with time; therefore the motion of the whole body may be represented by a particle. But when the body rotates or vibrates during translatory motion, then its motion can be represented by a point on the body that moves in the same way as that of a single particle subjected to the same external forces would move. A point in the system at which whole mass of the body is supposed to be concentrated is called centre of mass of the body. Therefore, if a system contains two or more particles, its translatory motion can be described by the motion of the centre of mass of the system.

Centre of mass of a two-particle system
Let us consider a system consisting of two particles of masses m1 and m2. P1 and P2 are their positions at time t and r1 and r2 are the corresponding distances from the origin O as shown in Fig. below. Then the velocity and acceleration of the particles are,

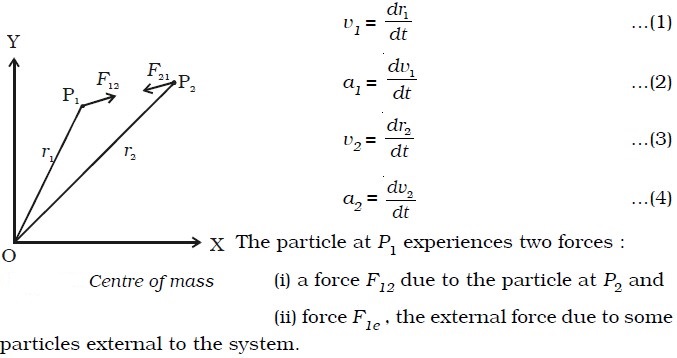
The particle at P1 experiences two forces :

● a force F12 due to the particle at P2 and
● force F1e , the external force due to some particles external to the system.

If F1 is the resultant of these two forces,
F1 = F12 + F1e …(5)

Similarly, the net force F2 acting on the particle P2 is,
F2 = F21 + F2e …(6)

where F21 is the force exerted by the particle at P1 on P2 By using Newton’s second law of motion,
F1 = m1a1 …(7)

and F2= m2a2 …(8)

Adding equations (7) and (8), m1a1 + m2a2 = F1 + F2

Substituting F1 and F2 from (5) and (6)

m1a1 + m2a2 = F12 + F1e+ F21 + F2e
By Newton’s third law, the internal force F12 exerted by particle at P2 on the particle at P1 is equal and opposite to F21, the force exerted by particle at P1 on P2.

(i.e) F12 = – F21 …(9)
∴ F = F1e+ F2e …(10)
[∵m1a1 +m2a2 = F ]

where F is the net external force acting on the system. The total mass of the system is given by,
M = m1+m2 …(11)

Let the net external force F acting on the system produces an acceleration aCM called the acceleration of the centre of mass of the system By Newton’s second law, for the system of two particles,
F = M aCM …(12)

From (10) and (12), M aCM = m1a1+m2a2 …(13)
Let RCM be the position vector of the centre of mass.

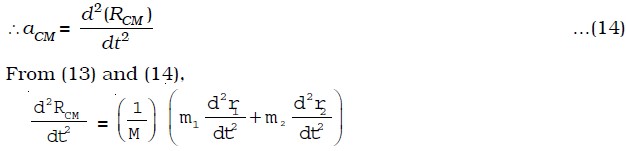
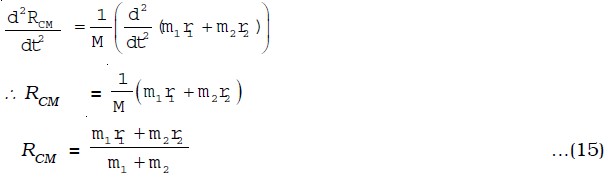
This equation gives the position of the centre of mass of a system comprising two particles of masses m1 and m2. If the masses are equal (m1 = m2), then the position vector of the centre of mass is,

RCM = r1 + r2 / 2                                                                    …(16)

which means that the centre of mass lies exactly in the middle of the line joining the two masses.

Centre of mass of a body consisting of n particles
For a system consisting of n particles with masses m1, m2, m3 … mn with position vectors r1, r2, r3…rn, the total mass of the system is,
M = m1 + m2 +m3 +………….+mn

The position vector RCM of the centre of mass with respect to origin O is given by

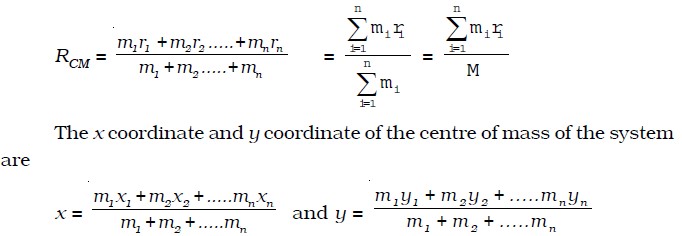
Example for motion of centre of mass
Let us consider the motion of the centre of mass of the Earth and moon system (Fig below). The moon moves round the Earth in a circular orbit and the Earth moves round the Sun in an elliptical orbit. It is more correct to say that the Earth and the moon both move in circular orbits about their common centre of mass in an elliptical orbit round the Sun.

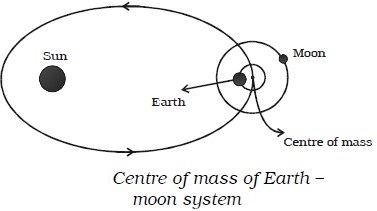
For the system consisting of the Earth and the moon, their mutual gravitational attractions are the internal forces in the system and Sun’s attraction on both the Earth and moon are the external forces acting on the centre of mass of the system.

Centre of gravity
A body may be considered to be made up of an indefinitely large number of particles, each of which is attracted towards the centre of the Earth by the force of gravity. These forces constitute a system of like parallel forces. The resultant of these parallel forces known as the weight of the body always acts through a point, which is fixed relative to the body, whatever be the position of the body. This fixed point is called the centre of gravity of the body. The centre of gravity of a body is the point at which the resultant of the weights of all the particles of the body acts, whatever may be the orientation or position of the body provided that its size and shape remain unaltered.

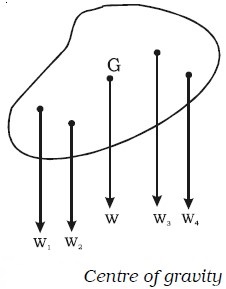
In the Fig. above, W1,W2,W3….. are the weights of the first, second, third, … particles in the body respectively. If W is the resultant weight of all the particles then the point at which W acts is known as the centre of gravity. The total weight of the body may be supposed to act at its centre of gravity. Since the weights of the particles constituting a body are practically proportional to their masses when the body is outside the Earth and near its surface, the centre of mass of a body practically coincides with its centre of gravity.

Equilibrium of bodies and types of equilibrium
If a marble M is placed on a curved surface of a bowl S, it rolls down and settles in equilibrium at the lowest point A (Fig. below a). This equilibrium position corresponds to minimum potential energy. If the marble is disturbed and displaced to a point B, its energy increases When it is released, the marble rolls back to A. Thus the marble at the position A is said to be in stable equilibrium. Suppose now that the bowl S is inverted and the marble is placed at its top point, at A (Fig.below b). If the marble is displaced slightly to the point C, its potential energy is lowered and tends to move further away from the equilibrium position to one of lowest energy. Thus the marble is said to be in unstable equilibrium. Suppose now that the marble is placed on a plane surface (Fig. below c). If it is displaced slightly, its potential energy does not change. Here the marble is said to be in neutral equilibrium.

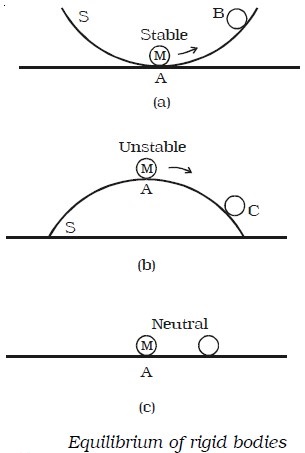
Equilibrium is thus stable, unstable or neutral according to whether the potential energy is minimum, maximum or constant.
We may also characterize the stability of a mechanical system by noting that when the system is disturbed from its position of equilibrium, the forces acting on the system may
● tend to bring back to its original position if potential energy is a minimum, corresponding to stable equilibrium.
● tend to move it farther away if potential energy is maximum, corresponding unstable equilibrium.
● tend to move either way if potential energy is a constant corresponding to neutral equilibrium.

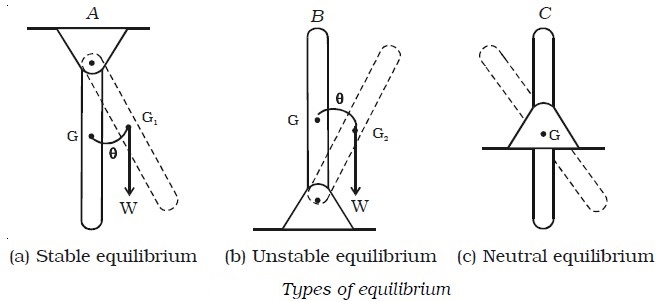
Consider three uniform bars shown in Fig. above a,b,c. Suppose each bar is slightly displaced from its position of equilibrium and then released. For bar A, fixed at its top end, its centre of gravity G rises to G1 on being displaced, then the bar returns back to its original position on being released, so that the equilibrium is stable.

For bar B, whose fixed end is at its bottom, its centre of gravity G is lowered to G2 on being displaced, then the bar B will keep moving away from its original position on being released, and the equilibrium is said to be unstable.

For bar C, whose fixed point is about its centre of gravity, the centre of gravity remains at the same height on being displaced, the bar will remain in its new position, on being released, and the equilibrium is said to be neutral.