Consider a body of mass m placed at P at a distance r from the centre of the Earth. Let the mass of the Earth be M.
When the mass m is at A at a distance x from Q, the gravitational force of attraction on it due to mass M is given by F = GM m /x^2
The work done in moving the mass m through a small distance dx from A to B along the line joining the two centres of masses m and M is
dw = –F.dx
Negative sign indicates that work is done against the gravitational field.
∴ dw = – 2GMm/x2 . dx
The gravitational potential energy of a mass m at a distance r from another mass M is defined as the amount of work done in moving the mass m from a distance r to infinity. The total work done in moving the mass m from a distance r to infinity is

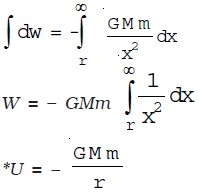
Gravitational potential energy is zero at infinity and decreases as the distance decreases. This is due to the fact that the gravitational force exerted on the body by the Earth is attractive. Hence the gravitational potential energy U is negative.

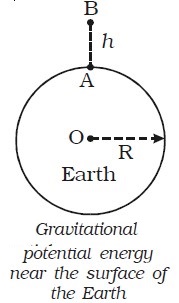
Gravitational potential energy near the surface of the Earth
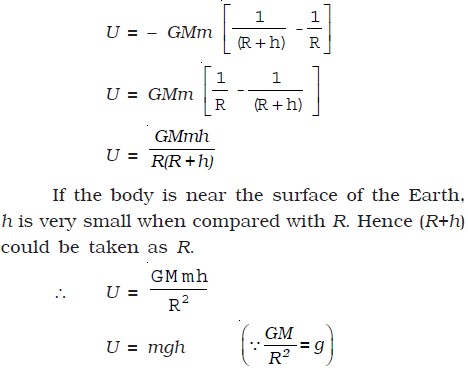
Let the mass of the Earth be M and its radius be R. Consider a point A on the surface of the Earth and another point B at a height h above the surface of the Earth. The work done in moving the mass m from A to B is U = UB − UA